Coxeter group
Within group theory, Coxeter groups are a kind of abstract group which have a close relationship with groups generated by geometric reflections. All Coxeter groups have a corresponding Coxeter-Dynkin diagram.
Definition[edit | edit source]
Coxeter groups can be defined in terms of a group presentation. A rank n Coxeter group is a group presentation generated by n involutions (i.e. for each generator, ρ i , we have the relation ρ 2
i = ε ), plus additional relations of the form:
where ρ i and ρ j are generators. Relations of this form are called braid relations.
Because the generators are involutions these relations can also be written as:
Coxeter matrix[edit | edit source]
Since each relation in the presentation of a Coxeter group (including the involution requirement on the generators) is of the form:
It is determined by the two generators it relates and the power k .
Thus the entire Coxeter group with n generators can be represented as an n by n symmetric matrix called the Coxeter matrix. Each row and column corresponds to a generator of the group, with the value of M i,j being the power k in the relation on the generators ρ i and ρ j . If there is no relation between to generators we put ∞ as their power in the matrix.
The Coxeter matrix is always symmetric, and its diagonal consists of 1s.
Coxeter-Dynkin diagrams[edit | edit source]
We can adapt the Coxeter matrix to a more concise format. We use the Coxeter matrix as the adjacency matrix for a edge-labeled graph. That is for an n ×n Coxeter matrix, M , we create a graph with n nodes connecting node i and j with an edge labeled M i,j ignoring the cases where i = j . Since the usually the majority of the entries in a given matrix are 2, we remove all edges labeled 2 to greatly simplify the resulting diagram. By convention we usually draw the diagram leaving edges labeled 3 unlabeled.
Examples[edit | edit source]
- The Coxeter matrix has the diagram





- The Coxeter matrix has the diagram







- The Coxeter matrix has the diagram
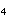



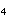
Linear Coxeter groups[edit | edit source]
A Coxeter group is linear if there exists an indexing of its generators such that all pairs of non-adjacent generators commute.
These groups are called linear because their Coxeter diagram can be drawn as a single line.
Non-adjacent distinguished generators of a regular polytope must commute, thus if the symmetry group of a regular polytope is a Coxeter group[note 1] that group is a linear Coxeter group.
See also[edit | edit source]
- Shephard groups, a generalization of linear Coxeter groups.
- C string groups, quotients of linear Coxeter groups and the automorphism groups of abstract regular polytopes.
- Artin-Tits groups, groups generated with braid relations closely related to Coxeter groups.
External links[edit | edit source]
- Wikipedia contributors. "Coxeter group".
- Weisstein, Eric W. "Coxeter Group" at MathWorld.
- nLab contributors. "Coxeter group" on nLab.
Notes[edit | edit source]
- ↑ This is the case for all planar polytopes, and many but not all abstract polytopes
| This article is a stub. You can help Polytope Wiki by expanding it. |


![{\displaystyle \left[{\begin{matrix}1&5&2\\5&1&3\\2&3&1\end{matrix}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/0b0520f644efe6d8ff53bdf4408468186e703768)
![{\displaystyle \left[{\begin{matrix}1&4&2&2\\4&1&3&2\\2&3&1&3\\2&2&3&1\end{matrix}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8e79b2ad9b1813195be8428a89b23bceb54ddd04)
![{\displaystyle \left[{\begin{matrix}1&4&2&3\\4&1&3&2\\2&3&1&4\\3&2&4&1\end{matrix}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/376bfaf9332402831db81a6ebca439ea0661a507)