Cube
| Cube | |
|---|---|
 | |
| Rank | 3 |
| Type | Regular |
| Notation | |
| Bowers style acronym | Cube |
| Coxeter diagram | x4o3o ( |
| Schläfli symbol | {4,3} |
| Tapertopic notation | 111 |
| Toratopic notation | III |
| Bracket notation | [III] |
| Conway notation | C |
| Stewart notation | P4 |
| Elements | |
| Faces | 6 squares |
| Edges | 12 |
| Vertices | 8 |
| Vertex figure | Triangle, edge length √2  |
| Petrie polygons | 4 skew hexagons |
| Measures (edge length 1) | |
| Circumradius | |
| Edge radius | |
| Inradius | |
| Volume | 1 |
| Dihedral angle | 90° |
| Height | 1 |
| Central density | 1 |
| Number of external pieces | 6 |
| Level of complexity | 1 |
| Related polytopes | |
| Army | Cube |
| Regiment | Cube |
| Dual | Octahedron |
| Petrie dual | Petrial cube |
| κ ? | Petrial tetrahedron |
| Conjugate | None |
| Abstract & topological properties | |
| Flag count | 48 |
| Euler characteristic | 2 |
| Surface | Sphere |
| Orientable | Yes |
| Genus | 0 |
| Skeleton | Q 3 |
| Properties | |
| Symmetry | B3, order 48 |
| Flag orbits | 1 |
| Convex | Yes |
| Net count | 11[1] |
| Nature | Tame |
The cube or hexahedron is one of the five Platonic solids. It has 6 square faces, joining 3 faces to a triangular vertex. It is the 3-dimensional hypercube.
It is the only Platonic solid that can tile 3-dimensional Euclidean space. This results in the cubic honeycomb. It also forms the cells of the 4D tesseract.
It is also the uniform square prism.
Vertex coordinates[edit | edit source]
Coordinates for the vertices of a cube of edge length 1, centered at the origin, are:
- .
Representations[edit | edit source]
A cube can be represented by the following Coxeter diagrams:
- x4o3o (




 ) (regular)
) (regular) - x2x4o (




 ) (generally a square prism)
) (generally a square prism) - x2x2x (




 ) (generally a cuboid)
) (generally a cuboid) - s2s4x (




 ) (generally a rectangular trapezoprism)
) (generally a rectangular trapezoprism) - x2s4s (




 )
) - x2s4x (




 )
) - p2p6o (
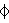

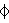

 ) (generally a triangular antitegum)
) (generally a triangular antitegum) - p2p3p (
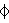

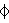

 )
) - xx4oo&#x (B2 axial, generally a square frustum)
- xx xx&#x (A1×A1 axial, rectangular frustum)
- oqoo3ooqo&#xt (A2 axial, generally a trigonal trapezohedron
- xx oqo&#xt (A1×A1, edge-first)
- oqooqo&#xt (A1 only)
- xxxx&#xr (A1 only, edge first)
- qo3oo3oq&#zx (A3 subsymmetry, hull of two tetrahedra)
- xx qo oq&#zx (rhombic prism)
In vertex figures[edit | edit source]
| Name | Picture | Schläfli symbol | Edge length |
|---|---|---|---|
| Icositetrachoron |  |
{3,4,3} | |
| Square tiling honeycomb |  |
{4,4,3} |
Variations[edit | edit source]
A cube can be considered as the prism product of three mutually orthogonal dyads with the same length. By adjusting the sizes of these edges, we can create variations with different symmetry. Further notable variations of the cube arise from taking subgroups of the full B3 symmetry.
All of these double as colorings of the cube, when their symmetry is transferred to the regular cube.
The most notable variations include:
- Square prism - two squares and 4 rectangles, identical vertices
- Cuboid or rectangular prism - 3 pairs of rectangles, identical vertices
- Rectangular trapezoprism - 2 rectangles and 4 isosceles trapezoids with digonal antiprism symmetry, identical vertices
- Triangular antitegum - 6 identical rhombic faces, trigonal antiprism symmetry
- Square frustum - 2 base squares of different sizes, 4 isosceles trapezoid sides
- Triangular gyrotegum: 6 identical tetragonal faces, chiral triangular prismatic symmetry
- Rectangular frustum - 2 different base rectangles, with 2 pairs of isosceles trapezoid sides, digonal pyramid symmetry
Related polyhedra[edit | edit source]
The cube can be augmented with a square pyramid to form the elongated square pyramid, which is a Johnson solid. If the opposite face is also augmented with a square pyramid, the result is the elongated square bipyramid.
A number of uniform polyhedron compounds are composed of cubes:
- Rhombihedron (5)
- Rhombihexahedron (3)
- Rhombisnub dishexahedron (6, with rotational freedom)
- An infinite number of prismatic compounds that are the prisms of compounds of squares
It is nontrivial to find a realizable polychoron with an odd number of cells, all of which are combinatorial cubes. The first such polychoron to be found has 16,533 cells. See cubical polytope.
External links[edit | edit source]
- Bowers, Jonathan. "Polyhedron Category 1: Regulars" (#2).
- Klitzing, Richard. "Cube".
- Quickfur. "The Cube".
- Hi.gher.Space Wiki Contributors. "Cube".
- Hartley, Michael. "{4,3}*48".
- Wedd, N. The cube
References[edit | edit source]
- ↑ Goldstone, Richard; Suzzi Valli, Robert (2016-04-18), Unfoldings of the Cube, doi:10.48550/arXiv.1604.05004 Check
|doi=value (help)















