Order-∞ square tiling
Jump to navigation
Jump to search
| Order-∞ square tiling | |
|---|---|
 | |
| Rank | 3 |
| Type | Regular, paracompact |
| Space | Hyperbolic |
| Notation | |
| Bowers style acronym | Asquat |
| Coxeter diagram | o∞o4x (    ) |
| Schläfli symbol | {4,∞} |
| Elements | |
| Faces | NM squares |
| Edges | 2NM |
| Vertices | 4N |
| Vertex figure | Apeirogon, edge length √2 |
| Measures (edge length 1) | |
| Circumradius | |
| Related polytopes | |
| Army | Asquat |
| Regiment | Asquat |
| Dual | Order-4 apeirogonal tiling |
| Abstract & topological properties | |
| Surface | Sphere |
| Orientable | Yes |
| Genus | 0 |
| Properties | |
| Symmetry | [∞,4] |
| Convex | Yes |
The order-∞ square tiling or infinite-order square tiling is a paracompact regular tiling of the hyperbolic plane. Infinitely many ideal squares join at each vertex. All vertices are ideal points at infinity.
The tiling can be alternated to produce the order-∞ apeirogonal tiling.
Representations[edit | edit source]
An order–∞ square tiling has the following Coxeter diagrams:
- o∞o4x () (full symmetry)
- o∞x4o4*a (
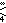
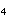) (squares of two types)
External links[edit | edit source]
- Klitzing, Richard. "asquat".
- Wikipedia contributors. "Infinite-order square tiling".
| truncations | ||||
|---|---|---|---|---|
| Name | OBSA | Schläfli symbol | CD diagram | Image |
| Order-4 apeirogonal tiling | squazat | {∞,4} | |  |
| Tetraapeirogonal tiling | tezt | r{∞,4} | |  |
| Order-∞ square tiling | azsquat | {4,∞} | | |
| Truncated order-4 apeirogonal tiling | tosquazat | t{∞,4} | |  |
| Small rhombitetraapeirogonal tiling | srotezt | rr{∞,4} | |  |
| Truncated order-∞ square tiling | tazsquat | t{4,∞} | |  |
| Great rhombitetraapeirogonal tiling | grotezt | tr{∞,4} | |  |
| Snub tetraapeirogonal tiling | sr{∞,4} |  |  | |