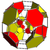
Truncated tesseract

Truncated tesseract
(OFF file)
Rank	4
Type	Uniform
Notation
Bowers style acronym	Tat
Coxeter diagram	x4x3o3o ()
Elements
Cells	16 tetrahedra, 8 truncated cubes
Faces	64 triangles, 24 octagons
Edges	32+96
Vertices	64
Vertex figure	Triangular pyramid, edge lengths 1 (base) and √2+√2 (sides)
Measures (edge length 1)
Circumradius	${\sqrt {\frac {5+3{\sqrt {2}}}{2}}}\approx 2.14973$
Hypervolume	${\frac {101+72{\sqrt {2}}}{6}}\approx 33.80390$
Dichoral angles	Tic–3–tet: 120°
	Tic–8–tic: 90°
Central density	1
Number of external pieces	24
Level of complexity	4
Related polytopes
Army	Tat
Regiment	Tat
Dual	Tetrakis hexadecachoron
Conjugate	Quasitruncated tesseract
Abstract & topological properties
Flag count	1536
Euler characteristic	0
Orientable	Yes
Properties
Symmetry	B4, order 384
Convex	Yes
Nature	Tame

The truncated tesseract, or tat, is a convex uniform polychoron that consists of 16 regular tetrahedra and 8 truncated cubes. 1 tetrahedron and three truncated cubes join at each vertex. As the name suggests, it can be obtained by truncating the tesseract.

As the truncated tesseract, it is the square member of an infinite family of isogonal truncated duoprisms, and could be called the truncated square duoprism.

Gallery

• 

  B4 Coxeter plane projection

• 

  Stereographic projection

• 

  Cross-sections

• 

  Net

Vertex coordinates

The vertices of a truncated tesseract of edge length 1 are given by all permutations of:

• $\left(\pm {\frac {1+{\sqrt {2}}}{2}},\,\pm {\frac {1+{\sqrt {2}}}{2}},\,\pm {\frac {1+{\sqrt {2}}}{2}},\,\pm {\frac {1}{2}}\right)$.

Representations

A truncated tesseract has the following Coxeter diagramms:

• x4x3o3o () (full symmetry)
• xwwx4xoox3oooo&#xt (B₃ symmetry, truncated cube-first)
• xwwxoooo3ooxwwxoo3ooooxwwx&#xt (A₃ axial, tetrahedron-first)
• wx3oo3xw *b3oo&#zx (D₄ symmetry)
• wx xw4xo3oo&#zx (B₃×A₁ symmetry)
• ox4wx xo4xw&#zx (B₂×B₂ symmetry, truncated square duoprism)
• xwww wxww wwxw wwwx&#zx (K₄ symmetry)

Semi-uniform variant

The truncated tesseract has a semi-uniform variant of the form x4y3o3o that maintains its full symmetry. This variant uses 16 tetrahedra of size y and 8 semi-uniform truncated cubes of form x4y3o as cells, with 2 edge lengths.

With edges of length a (surrounded by truncated cubes only) and b (of tetrahedra), its circumradius is given by ${\sqrt {\frac {2a^{2}+3b^{2}+3ab{\sqrt {2}}}{2}}}$ and its hypervolume is given by ${\frac {6a^{4}+72a^{2}b^{2}+23b^{4}+(24a^{3}b+48ab^{3}){\sqrt {2}}}{6}}$.

It has coordinates given by all permutations of:

• $\left(\pm {\frac {a+b{\sqrt {2}}}{2}},\,\pm {\frac {a+b{\sqrt {2}}}{2}},\,\pm {\frac {a+b{\sqrt {2}}}{2}},\,\pm {\frac {a}{2}}\right)$.

Related polychora

Uniform polychoron compounds composed of truncated tesseracts include:

• Truncated great icositetrachoron (3)
• Truncated great stellated tetracontoctachoron (6)
• Truncated dodecahedronary cubichoron (75)

External links

• Bowers, Jonathan. "Category 2: Truncates" (#19).

• Bowers, Jonathan. "Tessic Isogonals".

• Klitzing, Richard. "tat".
• Quickfur. "The Truncated Tesseract".

• Wikipedia contributors. "Truncated tesseract".